![Alexander Shemetev's photo [Alexander Shemetev]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/alexshemetev300dpi.jpg)
Working-out the self-model of Alexander Shemetev for firms" bankruptcy forecasting based on synthesis of 42-years experience of Ghent University
![Sofie (Sofia) Balcaen's photo [Sofie (Sofia) Balcaen]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/balcaens.jpg)
![Hubert Ooghe's photos [Hubert Ooghe]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/oogheh.jpg)
![Eric Verbaere's photo [Eric Verbaere ]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/verbaeree.jpg)
![LOGIT regression []](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/353.jpg) (353)
(353)
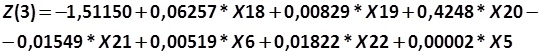
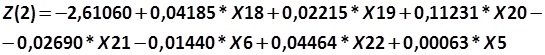
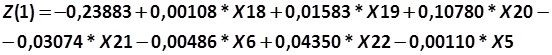
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/365.jpg)
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/366.jpg)
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/367.jpg)
|
|
||
Working-out the self-model of Alexander Shemetev for firms" bankruptcy forecasting based on synthesis of 42-years experience of Ghent University. Author's method of Alexander Shemetev for calculating the probability of failure and term prior to the bankruptcy, based on Ghent University studies. | ||
![Alexander Shemetev's photo [Alexander Shemetev]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/alexshemetev300dpi.jpg)
![Sofie (Sofia) Balcaen's photo [Sofie (Sofia) Balcaen]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/balcaens.jpg)
![Hubert Ooghe's photos [Hubert Ooghe]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/oogheh.jpg)
![Eric Verbaere's photo [Eric Verbaere ]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/verbaeree.jpg)
![LOGIT regression []](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/353.jpg) (353)
(353)
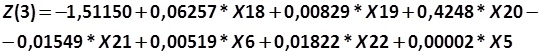
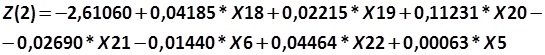
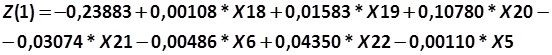
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/365.jpg)
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/366.jpg)
![A new method of Christine Zavgren [Christine Zavgren, Ghent University]](/img/s/shemetew_a_a/alexandershemetevworking-outtheself-modelofalexandershemetevforfirmsbankruptcyforecasting/367.jpg)